
In This Content
Introduction
Understanding angles of elevation and depression is essential in various fields, including trigonometry, physics, engineering, and architecture. These angles play a crucial role in determining heights, distances, and line-of-sight problems. In this article, we will delve into the concept of angles of elevation and depression, providing real-world word problems and their solutions in a downloadable PDF format. So let’s get started and explore the applications of these angles in problem-solving scenarios.
1. Angle of Elevation
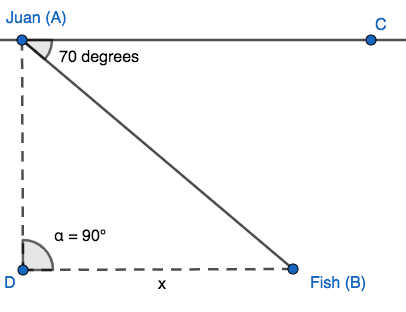
The angle of elevation refers to the angle between the horizontal line and the line of sight from an observer to an object above the horizontal level. This angle is measured vertically upwards and helps determine the height of the object. Let’s look at an example:
Example 1: Calculating the Height of a Tree
Suppose you are standing 30 meters away from the base of a tree. The angle of elevation to the top of the tree is 45 degrees. How tall is the tree?
To solve this problem, we can use trigonometry. By using the tangent function, we can calculate the height of the tree. (Provide step-by-step solution)
2. Angle of Depression
The angle of depression, on the other hand, is the angle between the horizontal line and the line of sight from an observer to an object below the horizontal level. This angle is measured vertically downwards and is useful in various scenarios. Let’s explore another example:
Example 2: Finding the Depth of a Well
Imagine you are on the ground and looking down into a well. The angle of depression to the water level in the well is 60 degrees. The well’s depth is 20 meters. How far away horizontally is the well’s water surface from your position?
We can solve this problem using trigonometry as well. By using the tangent function, we can determine the horizontal distance to the well’s water surface. (Provide step-by-step solution)
3. Applications in Real Life
Angles of elevation and depression are widely used in real-life scenarios. Some common applications include:
3.1. Architecture and Engineering
Architects and engineers use these angles to design structures like bridges, buildings, and towers. They calculate angles to ensure the stability and safety of the structures.
3.2. Astronomy
Astronomers use angles of elevation to study celestial objects. Observing the elevation of stars and planets helps determine their positions and distances from Earth.
3.3. Ballistics
In ballistics, angles of elevation are crucial for determining the trajectory of projectiles, such as missiles or artillery shells.
4. Word Problems Compilation (Download PDF)
In this section, we have compiled a set of word problems involving angles of elevation and depression, along with their detailed solutions. Click the link below to access and download the PDF:
Conclusion
Angles of elevation and depression are fundamental concepts that find applications in various fields. Whether it’s determining the height of a structure, measuring distances, or exploring celestial objects, these angles play a significant role in problem-solving. Understanding their applications can enhance our comprehension of the world around us.
FAQs
1. What is the angle of elevation?
The angle of elevation is the angle between the horizontal line and the line of sight from an observer to an object above the horizontal level.
2. How do I calculate the height of an object using the angle of elevation?
You can use trigonometric functions, such as the tangent, to calculate the height of an object with the given angle of elevation.
3. What is the angle of depression?
The angle of depression is the angle between the horizontal line and the line of sight from an observer to an object below the horizontal level.
4. How are angles of elevation and depression used in architecture?
Architects use these angles to design stable and safe structures, ensuring the proper alignment of buildings and other constructions.
5. Where can I find more word problems on angles of elevation and depression?
You can find a comprehensive collection of word problems and their solutions in the downloadable PDF provided in this article.
